Separable algebras

The man who knew elliptic integrals, prime number theorems, and black holes »

Separable algebras

March 27, 2016 by Qiaochu Yuan

Let $k$ be a commutative ring and let $A$ be a $k$ -algebra. In this post we’ll investigate a condition on $A$ which generalizes the condition that $A$ is a finite separable field extension (in the case that $k$ is a field). It can be stated in many equivalent ways, as follows. Below, “bimodule” always means “bimodule over $k$ .”

Definition-Theorem: The following conditions on $A$ are all equivalent, and all define what it means for $A$ to be a separable $k$ -algebra:

$A$ is projective as an $(A, A)$ -bimodule (equivalently, as a left $A \otimes_k A^{op}$ -module).
The multiplication map $A \otimes_k A^{op} \ni (a, b) \xrightarrow{m} ab \in A$ has a section as an $(A, A)$ -bimodule map.
$A$ admits a separability idempotent: an element $p \in A \otimes_k A^{op}$ such that $m(p) = 1$ and $ap = pa$ for all $a \in A$ (which implies that $p^2 = p$ ).

(Edit, 3/27/16: Previously this definition included a condition involving Hochschild cohomology, but it’s debatable whether what I had in mind is the correct definition of Hochschild cohomology unless $k$ is a field or $A$ is projective over $k$ . It’s been removed since it plays no role in the post anyway.)

When $k$ is a field, this condition turns out to be a natural strengthening of the condition that $A$ is semisimple. In general, loosely speaking, a separable $k$ -algebra is like a “bundle of semisimple algebras” over $\text{Spec } k$ .

Proofs that the above conditions are equivalent

$1 \Leftrightarrow 2$ : the multiplication map

$\displaystyle A \otimes_k A^{op} \ni a \otimes b \xrightarrow{m} ab \in A$

is an epimorphism of $(A, A)$ -bimodules, so if $A$ is projective as an $(A, A)$ -bimodule then it splits, meaning it has a section. Conversely, since $A \otimes_k A^{op}$ is a free $(A, A)$ -bimodule, if this map has a section then $A$ is a retract of a free $(A, A)$ -bimodule, hence is projective.

$2 \Leftrightarrow 3$ : a section of the multiplication map, as above, is determined by what it does to $1 \in A$ ; let’s call the image $p = \sum a_i \otimes b_i$ , and abuse terminology by identifying $p$ with the section it defines. What does it mean for $p$ to split the multiplication map? As a splitting, it must satisfy

$\displaystyle m(p) = \sum a_i b_i = 1 \in A$

since it’s the image of $1$ . Second, as an $(A, A)$ -bimodule map, it must satisfy $ap = pa$ for all $a \in A$ , since $a \cdot 1 = 1 \cdot a$ in $A$ . (In fact $A$ is the free $(A, A)$ -bimodule on a generator with this property.) These conditions together imply that

$\displaystyle p^2 = \sum_i a_i p b_i = \left( \sum_i a_i b_i \right) p = p$

hence that $p$ is an idempotent, as the name “separability idempotent” suggests. Hence a splitting of the multiplication map is the same data as a separability idempotent, which is in fact an idempotent.

This concludes the proof. $\Box$

A note on $A \otimes_k A^{op}$

Above we chose to write the source of the multiplication map as $A \otimes_k A^{op}$ to emphasize that it is the free $A \otimes_k A^{op}$ -module, or equivalently the free $(A, A)$ -bimodule, on a generator. However, it can just as well be written $A \otimes_k A$ , provided that we remember that the natural $(A, A)$ -bimodule structure on this is given by left multiplication on the first copy of $A$ and right multiplication on the second copy of $A$ . (Also, when we think of the separability idempotent as an idempotent, we really have in mind the algebra structure on $A \otimes_k A^{op}$ .) This is how we’ll write things down in examples below.

Some examples

Example. $k$ itself is a separable $k$ -algebra, since it is even free as a $k \otimes_k k^{op} \cong k$ -module.

Example. The matrix algebra $M_n(k)$ is a separable $k$ -algebra. We can prove this explicitly by writing down a separability idempotent. Letting $e_{ij}, 1 \le i, j \le n$ be the usual basis of $M_n(k)$ (with relations $e_{ij} e_{jk} = e_{ik}$ , and all other multiplications zero), set

$\displaystyle p = \sum_i e_{ij} \otimes e_{ji} \in M_n(k) \otimes M_n(k)$

where $j$ is fixed. We have

$\displaystyle m(p) = \sum_i e_{ij} e_{ji} = \sum_i e_{ii} = 1$

and

$e_{ab} p = \sum_i e_{ab} e_{ij} \otimes e_{ji} = e_{aj} \otimes e_{jb}$

while

$\displaystyle p e_{ab} = \sum_i e_{ij} \otimes e_{ji} e_{ab} = e_{aj} \otimes e_{jb}$

so $p$ is a separability idempotent.

Example. If $G$ is a finite group whose order $|G|$ is invertible in $k$ , then the group algebra $k[G]$ is a separable $k$ -algebra. Again we can prove this explicitly by writing down a separability idempotent. Set

$\displaystyle p = \frac{1}{|G|} \sum_g g \otimes g^{-1} \in k[G] \otimes k[G]$ .

We have

$\displaystyle m(p) = \frac{1}{|G|} \sum_g g g^{-1} = 1 \in k[G]$

and, for any $h \in G$ ,

$\displaystyle \begin{aligned} hp &= \frac{1}{|G|} \sum_g hg \otimes g^{-1} \\ &= \frac{1}{|G|} \sum_g g \otimes (h^{-1} g)^{-1} \\ &= \frac{1}{|G|} \sum_g g \otimes g^{-1} h \\ &= ph \end{aligned}$

where in the second line we made the substitution $hg \mapsto g$ . So $p$ is a separability idempotent.

To get some more examples it will be convenient to use the following lemma.

Lemma: If $A \otimes_k A^{op}$ is semisimple, then $A$ is separable over $k$ .

Proof. A ring is semisimple iff every module over it is projective. So if $A \otimes_k A^{op}$ is semisimple, then in particular $A$ is a projective $A \otimes_k A^{op}$ -module. $\Box$

Corollary: If $L$ is a finite separable extension of a field $k$ (in the usual sense), then $L$ is separable over $k$ (in the above sense).

Proof. By the primitive element theorem, $L = k[x]/f(x)$ for some irreducible separable polynomial $f(x)$ . Hence

$\displaystyle L \otimes_k L^{op} \cong L[x]/f(x) \cong \prod_i L[x]/f_i(x)$

where $f_i(x)$ are the irreducible factors of $f(x)$ over $L[x]$ (it has at least two, since by definition $L$ contains a root of $f(x)$ ). This is a finite product of fields and hence semisimple, so by the lemma we conclude that $L$ is separable. $\Box$

Over a field, this gives another way to prove that the matrix algebras $M_n(k)$ and the group algebras $k[G]$ (where $|G|$ is invertible in $k$ ) are separable: $M_n(k) \otimes_k M_n(k)^{op} \cong M_{n^2}(k)$ is semisimple, and so is $k[G] \otimes_k k[G]^{op} \cong k[G \times G^{op}]$ . But the proofs via writing down separability idempotents work for much more general base rings $k$ .

Some general lemmas

Lemma: $A$ is a separable $k$ -algebra iff $A^{op}$ is.

Proof. The opposite of a separability idempotent for $A$ is a separability idempotent for $A^{op}$ .

More explicitly, suppose $p = \sum a_i \otimes b_i \in A \otimes_k A^{op}$ is a separability idempotent for $A$ . Then $p^{op} = \sum b_i \otimes a_i \in A^{op} \otimes A$ is a separability idempotent for $A^{op}$ . $\Box$

Lemma: If $A$ and $B$ are separable $k$ -algebras, then so is $A \times B$ .

Proof. The sum of separability idempotents for $A$ and $B$ is a separability idempotent for $A \times B$ .

More explicitly, recall that tensor product distributes over finite products for algebras. (In the commutative case this means that product distributes over finite coproducts for affine schemes.) Hence

$(A \times B) \otimes_k (A \times B) \cong (A \otimes_k A) \times (A \otimes_k B) \times (B \otimes_k A) \times (B \otimes_k B)$

and this is even an isomorphism of $(A \times B, A \times B)$ -bimodules, respecting the multiplication map down to $A \times B$ . From this it’s not hard to verify that if $p_A \in A \otimes_k A$ is a separability idempotent for $B$ , and $p_B \in B \otimes_k B$ is a separability idempotent for $B$ , then $p_A + p_B \in (A \otimes_k A) \times (B \otimes_k B)$ , included into the above, is a separability idempotent for $A \times B$ . $\Box$

Lemma: If $A$ and $B$ are separable $k$ -algebras, then so is $A \otimes_k B$ .

Proof. The tensor product of separability idempotents for $A$ and $B$ is a separability idempotent for $A \otimes_k B$ . $\Box$

Lemma: If $A$ is a separable $k$ -algebra, then the base change $A \otimes_k K$ is a separable $K$ -algebra, for any commutative $k$ -algebra $K$ . (Hence separability is a geometric property in the strong sense that it is preserved by arbitrary base change.)

Proof. A separability idempotent for $A$ remains a separability idempotent for $A \otimes_k K$ . $\Box$

Note that this is not true for semisimple algebras, since an inseparable extension of the ground field is a counterexample.

Lemma: Any quotient of a separable algebra is separable.

Proof. A separability idempotent for $A$ remains a separability idempotent for any quotient of $A$ . $\Box$

Corollary: Two $k$ -algebras $A, B$ are separable over $k$ if and only if $A \times B$ is separable over $k$ .

Proof. In one direction, if $A, B$ are separable, then so is $A \times B$ . In the other, if $A \times B$ is separable, then $A, B$ are quotients of it, hence are also separable. $\Box$

Lemma: Separability is Morita invariant: if $A$ and $B$ are Morita equivalent over $k$ , then $A$ is separable over $k$ iff $B$ is.

Proof. By the Eilenberg-Watts theorem, the category of $(A, A)$ -bimodules is equivalent (even monoidally) to the category of cocontinuous $k$ -linear endofunctors of $\text{Mod}(A)$ . Among these, the bimodule $A$ itself represents the identity functor. Hence separability is equivalent to the condition that the identity is projective, and since this condition can be stated entirely in terms of $\text{Mod}(A)$ it is Morita invariant. $\Box$

Lemma: If $R$ is a commutative $k$ -algebra and $A$ is an $R$ -algebra such that 1) $R$ is separable over $k$ and 2) $A$ is separable over $R$ , then $A$ is separable over $k$ .

Proof. By hypothesis, the multiplication maps $R \otimes_k R^{op} \to R$ and $A \otimes_R A^{op} \to A$ split as bimodule maps, and we want to know that the same is true of the multiplication map $A \otimes_k A^{op} \to A$ . (Note that we are writing $R^{op}$ even though $R$ is commutative and so canonically isomorphic to its opposite; we don’t want to use this isomorphism.) If we write

$\displaystyle A \otimes_k A^{op} \cong A \otimes_R (R \otimes_k R^{op}) \otimes_{R^{op}} A^{op}$

then we can factor the multiplication map as a composite of two maps we know how to split, namely

$\displaystyle A \otimes_R (R \otimes_k R^{op}) \otimes_{R^{op}} A^{op} \to A \otimes_R A^{op} \to A$

where the first map applies the multiplication map $R \otimes_k R^{op} \to R$ between the two copies of $A$ and the second map is the multiplication map $A \otimes_R A^{op} \to A$ . (A similar argument can be used to show that the tensor product of separable algebras is separable.) $\Box$

Classification over a field

We now classify separable $k$ -algebras when $k$ is a field.

Lemma: If $A$ is separable over a field $k$ , then $A$ is semisimple.

Proof. Any $(A, A)$ -bimodule $M$ describes a cocontinuous $k$ -linear functor $\text{Mod}(A) \to \text{Mod}(A)$ as follows:

$\displaystyle \text{Mod}(A) \ni V \mapsto M \otimes_A V \in \text{Mod}(A)$ .

The bimodule $A$ represents the identity functor, while the free bimodule $A \otimes_k A$ represents the functor

$\displaystyle \text{Mod}(A) \ni V \mapsto A \otimes_k V \ni \text{Mod}(A)$ .

Consequently, the multiplication map $A \otimes_k A \xrightarrow{m} A$ represents the natural transformation

$\displaystyle A \otimes_k V \to V$

given by the action of $A$ . Now, if $A$ is separable, then this natural transformation splits, and in particular all of these action maps split. If $k$ is a field, then $V$ is a free $k$ -module, so $A \otimes_k V$ is a free $A$ -module. This means that every $A$ -module $V$ is a retract of a free $A$ -module, hence is projective, and so $A$ is semisimple as desired. $\Box$

Corollary: If $A$ is separable over a field $k$ , then $A$ is a finite product of matrix algebras over division algebras $D_i$ over $k$ , all of which must also be separable.

Proof. Since $A$ is semisimple, Artin-Wedderburn implies that $A \cong \prod_{i=1}^n M_{d_i}(D_i)$ for some division rings $D_i$ over $k$ . The lemmas we proved above imply that $A \times B$ is separable iff $A, B$ are separable and that $A$ is separable iff $M_d(A)$ is separable, so any such product is separable iff each $D_i$ is separable. $\Box$

Corollary: If $A$ is separable over a field $k$ , then $A$ is geometrically semisimple: $A \otimes_k L$ is semisimple for every field extension $k \to L$ .

Proof. We know that separability is geometric (preserved by base change), so $A \otimes_k L$ is separable over $L$ for every $L$ . If $L$ is a field extension, then the above lemma implies that $A \otimes_k L$ is also semisimple. $\Box$

(Edit, 2/6/18: Previously this section contained an incorrect proof that a separable algebra is finite-dimensional, as pointed out by Antoine in the comments.)

Corollary: An algebra $A$ over a field $k$ is separable over $k$ iff $A \otimes_k A^{op}$ is semisimple.

Proof. Above we showed that if $A \otimes_k A^{op}$ is semisimple, then $A$ is separable over $k$ (since every module, and in particular $A$ , is projective over $A \otimes_k A^{op}$ ). Conversely, we also showed that tensor products and opposites of separable algebras are separable, so if $A$ is separable over $k$ then so is $A \otimes_k A^{op}$ , and so it must also be semisimple. $\Box$

At this point we’ve reduced to looking for division algebras $D$ over $k$ such that $D \otimes_k D^{op}$ is semisimple. We’ll find them by inspecting their centers $Z(D)$ , which are field extensions of $k$ .

Lemma: Let $A, B$ be algebras over a field $k$ , and let $Z(-)$ denote the center. Then $Z(A \otimes_k B) \cong Z(A) \otimes_k Z(B)$ .

Proof. Suppose $z = \sum a_i \otimes b_i \in Z(A \otimes_k B)$ is central. This is equivalent to the condition that

$\displaystyle [z, a \otimes 1] = \sum [a_i, a] \otimes b_i = 0$

for all $a \in A$ and

$\displaystyle [z, 1 \otimes b] = \sum a \otimes [b_i, b] = 0$ .

for all $b \in B$ . Since we’re working over a field, we can assume WLOG that the $a_i$ and $b_i$ are linearly independent in $A$ and $B$ respectively, from which it follows that these conditions hold if and only if $[a_i, a] = [b_i, b] = 0$ for all $i, a, b$ . Hence $z \in Z(A) \otimes_k Z(B)$ , which (again, since we’re working over a field) is naturally a subalgebra of $Z(A \otimes_k B)$ , and so must be the entire thing. $\Box$

Lemma: If $A$ is a separable algebra over a field $k$ , then so is its center $Z(A)$ .

Proof. We know that $A$ is separable iff $A \otimes_k A^{op}$ is semisimple. By the above lemma, we have

$\displaystyle Z(A \otimes_k A^{op}) \cong Z(A) \otimes_k Z(A)^{op}$

and since the center of a semisimple algebra is (a finite product of fields, hence) semisimple, it follows that $Z(A) \otimes_k Z(A)^{op}$ is semisimple, hence (by another lemma) that $Z(A)$ is separable. $\Box$

Theorem: Let $L$ be a field extension of a field $k$ . The following conditions are equivalent:

$L$ is a finite separable extension of $k$ (in the usual sense).
$L$ is a separable $k$ -algebra (in the above sense).
$L$ is geometrically semisimple: $L \otimes_k L'$ is semisimple for all field extensions $k \to L'$ .
$L \otimes_k \bar{k}$ is a finite product of copies of $\bar{k}$ .

(Edit, 2/7/18: The statement of this result has been modified to be correct but, because of the error above, the proof is incomplete. In particular the proofs of $3 \Rightarrow 4$ and $4 \Rightarrow 1$ below are incomplete. I don’t know when I’ll get around to completing them, unfortunately.)

Proof. $1 \Rightarrow 2$ : we proved this above from the primitive element theorem.

$2 \Rightarrow 3$ : follows from a lemma above.

$3 \Rightarrow 4$ : set $L' = \bar{k}$ .

$4 \Rightarrow 1$ : any $\alpha \in L$ generates a $\bar{k}$ -subalgebra of $L \otimes_k \bar{k}$ isomorphic to $\bar{k}[\alpha]/f(\alpha)$ where $f$ is the minimal polynomial of $\alpha$ . If $L \otimes_k \bar{k}$ is semisimple, it is a finite product of copies of $\bar{k}$ , hence so is every $\bar{k}$ -subalgebra of it. And $\bar{k}[\alpha]/f(\alpha)$ is a finite product of copies of $\bar{k}$ iff $f$ is separable over $k$ . $\Box$

Corollary: If a division algebra $D$ over a field $k$ is separable over $k$ , then $D$ is finite-dimensional over $k$ , and its center $Z(D)$ is a finite separable extension of $k$ (in the usual sense).

This necessary condition in fact turns out to be sufficient. We need one more lemma to prove this, which is the following.

Theorem: Let $A$ be a central simple algebra over a field $L$ : that is, $A$ is a finite-dimensional simple $L$ -algebra with center $L$ . Then $A \otimes_L A^{op} \cong M_n(L)$ , where $n = \dim_L A$ .

Proof. $A \otimes_L A^{op}$ naturally acts on $A$ ( $A$ acting from the left, $A^{op}$ acting from the right). Simplicity of $A$ means that $A$ has no nontrivial two-sided ideals, or equivalently has no nontrivial $A \otimes_L A^{op}$ -submodules, hence $A$ is simple as an $A \otimes_L A^{op}$ -module. Its endomorphism ring is the center $Z(A) \cong L$ , and as a module over this endomorphism ring, $A \cong L^n$ . Hence the natural action of $A \otimes_L A^{op}$ on $A$ gives a map

$\displaystyle A \otimes_L A^{op} \to \text{End}_L(A) \cong M_n(L)$

and we want this map to be a bijection. But it is a surjection by the Jacobson density theorem, hence a bijection since both sides have dimension $n^2$ over $L$ . $\Box$

Corollary: A central simple algebra over a field $L$ is separable over $L$ .

Corollary: A finite-dimensional division algebra $D$ over a field $k$ whose center $Z(D)$ is a finite separable extension of $k$ is separable over $k$ .

Proof. We now know that $D$ is separable over $Z(D)$ , and that $Z(D)$ is separable over $k$ . By a lemma, it follows that $D$ is separable over $k$ . $\Box$

Corollary: The separable algebras over a field $k$ are precisely the finite products of matrix algebras over finite-dimensional division algebras over $k$ whose centers are separable extensions of $k$ .

Over a perfect field, the last condition is automatic, so this just says that the separable algebras over $k$ are precisely the finite-dimensional semisimple $k$ -algebras.

Posted in math.CT, math.NT, math.RA | 4 Comments

4 Responses

on February 23, 2019 at 5:45 pm | Reply Kursat

I think in the matrix algebra case you need to divide separability idempotent by n. For example, take $i=1$ and consider $e_{11}$ in the multiplication. For $n$ different $j$ values you get $n$ many $e_{11}$. In particular, $n$ must be divisible in $k$.
- on March 22, 2019 at 12:43 pm | Reply Qiaochu Yuan
  
  Thanks for the comment!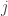 $j$ is fixed and I’m not summing over it.
on February 1, 2018 at 8:41 am | Reply Antoine

I don’t understand the sentence “(since there are no nontrivial division algebras over an algebraically closed field)” in one of the corollaries of the classification of separable algebras.

I am not at all an expert of this (this is why I read your blog) but I believe there are nontrivial division algebras over algebraically closed fields, but they must be infinite dimensional.

More simply, if k is algebraically closed, A=k(t) gives a semi-simple k-algebra which is not finite dimensional over k.

Am I making a mistake ?
- on February 3, 2018 at 5:58 pm | Reply Qiaochu Yuan
  
  You’re right. It looks like the argument you’re quoting needs to be repaired; $k(t)$ is not geometrically semisimple because it’s not semisimple after base change to $k(t)$ , but I need an additional argument to establish this sort of thing.

Comments RSS

	ZW on The double commutant theo…
	auto on More about the Schrödinger equ…
	Jaromir Kuben on Meditation on the Sylow theore…
	Qiaochu Yuan on Meditation on the Sylow theore…
	Qiaochu Yuan on Some remarks on the infinitude…

Annoying Precision

"A good stock of examples, as large as possible, is indispensable for a thorough understanding of any concept, and when I want to learn something new, I make it my first job to build one." – Paul Halmos